The Slope Intercept Form of the Equation of a Straight Line
Consider the straight line $$l$$. Let $$P\left( {x,y} \right)$$ be any point on the given line $$l$$. Suppose that $$\alpha $$ is the inclination of the line $$l$$ as shown in the given diagram, i.e. $$\tan \alpha = m$$
Take $$c$$ as a $$Y$$-intercept of the straight line because it cuts the $$Y$$-axis at the point $$Q\left( {0,c} \right)$$, i.e. $$OQ = c = Y$$-intercept.
From point $$P$$ draw $$PM$$ perpendicular to the $$X$$-axis, and from $$Q$$ draw $$QR$$ perpendicular to the $$PM$$.
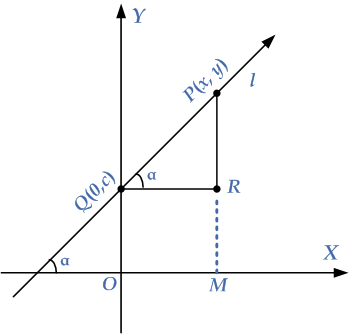
Now from the given diagram, consider the triangle $$\Delta PQR$$, i.e. $$m\angle RQP = \alpha $$.
By the definition of slope we take
\[\begin{gathered} \tan \alpha = \frac{{PR}}{{QR}} = \frac{{PM – RM}}{{OM}} \\ \Rightarrow \tan \alpha = \frac{{PM – OQ}}{{OM}} \\ \Rightarrow \tan \alpha = \frac{{y – c}}{x} \\ \end{gathered} \]
Now by the definition we can use $$m$$ instead of $$\tan \alpha $$, and we get
\[\begin{gathered} \Rightarrow m = \frac{{y – c}}{x} \\ \Rightarrow mx = y – c \\ \end{gathered} \]
\[\boxed{y = mx + c}\]
This is the equation of a straight line having the slope $$m$$ and Y-intercept $$c$$.
NOTE: It may be noted that if the line passes through the origin $$\left( {0,0} \right)$$, then take the $$Y$$-intercept is equal to zero i.e. $$c = 0$$, so the equation of a straight line becomes $$y = mx$$.
Example: Find the equation of a straight line having the slope $$3$$ and $$Y$$-intercept equal to 8.
Here we have slope $$m = 3$$ and $$Y$$-intercept $$c = 8$$
Now using the formula of straight line having the slope and $$Y$$-intercept
\[y = mx + c\]
Substitute the above values in the formula to get the equation of a straight line
\[y = 3x + 8\]
\[3x – y + 8 = 0\]
This is the required equation of a straight line.
