The Area of Certain Geometrical Figures

The area of certain geometrical figures refers to a number that in some way measures the size of the region enclosed by the figure. The area of a triangle is the product of its length and width, and the area of a triangle is half the product of the lengths of the base and the altitudes.

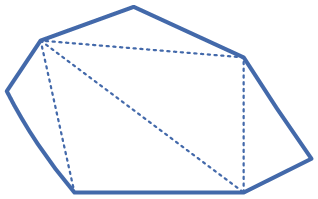
The area of a polygon may be defined as the sum of the areas of triangles into which it is decomposed, and it can be proved that the area thus obtained is independent of how the polygon is decomposed into triangles, as shown in the figure.

However, how do we define the area of a region in a plane if the region is bounded by a curve? Are we even certain that such a region has an area?