Completely Randomized Design

A completely randomized (CR) design, which is the simplest type of the basic designs, may be defined as a design in which the treatments are assigned to experimental units completely at random. That is, the randomization is done without any restrictions. The design is completely flexible, i.e., any number of treatments and any number of units per treatment may be used. Moreover, the number of units per treatment need not be equal. A completely randomized design is considered to be most useful in situations where (i) the experimental units are homogeneous, (ii) the experiments are small such as laboratory experiments, and (iii) some experimental units are likely to be destroyed or fail to respond.

Experimental Layout

The layout of an experiment is the actual placement of the treatments of the experimental units, which may pertain to time, space or type of material. Suppose we have the $$k – $$ treatment and the experimental material is divided into $$n$$ experimental units. We shall then assign the $$k – $$ treatments at random to the $$n$$ experimental units in such a way that the treatment $${\tau _j} = \left( {i = 1,2, \ldots ,k} \right)$$is applied $${r_j}$$times, with$$\sum {r_j} = n$$.When each treatment is applied the same number of times, then$${r_1} = {r_2} = \cdots = {r_k} = r$$ and $$\sum {r_j} = rk = n$$. Usually, each treatment is applied (or replicated) an equal number of times.
An example of the experimental layout for a completely randomized design (CR) using four treatments $$A,B,C$$ and $$D$$,each repeated $$3$$ times, is given below:

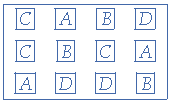
The result or response of a treatment which may be a real yield, i.e. weight gain, the ability, etc., is generally called yield and is represented by the letter $$Y$$.